Fyneman exercise I ch29-2

2.

Four identical dipole radiators are aligned parallel to one another and are equally spaced along a line at a distance $2.50 cm$ apart. They are driven at a frequency of $3.00 \times 10^9 sec^{-1}$ and are phased so that, starting from one end, each successive dipole lags the preceding one by $90^{\circ}$. Find the intensity pattern of the radiation at a great distance in the equatorial plane (perpendicular to the dipole axes), and sketch this function in polar coordinates. Such a diagram is called the radiation pattern of an antenna system.

My ans.

$d=2.50 \times 10^{-2} m$
$f=3.00 \times 10^9 sec^{-1}$
$c=3.00 \times 10^8 m\cdot sec^{-1}$
At fixed point in equatorial plane with $\theta$ angle from dipole radiators aligned line,
Let phase diff each adjacent dipole = $\phi$:

$\phi=kd\cos{\theta} - \frac{\pi}{2}$
, where $k=2\pi \frac{f}{c}=20\pi \ rad/m$.
$\therefore \phi = 20\pi\times 2.50 \times 10^{-2} \times \cos{\theta} - \frac{\pi}{2}=\frac{\pi}{2}\cos{\theta} - \frac{\pi}{2} = \frac{\pi}{2}(\cos{\theta}-1) \ rad$

first method

Field,
$E=E_0\{ \cos{\omega t} + \cos{(\omega t + \phi)} + \cos{(\omega t + 2\phi)} + \cos{(\omega t + 3\phi)}\}$
Using complex,
$E=E_0Re\{ e^{i\omega t} + e^{i(\omega t +\phi)} + e^{i(\omega t +2\phi)} + e^{i(\omega t +3\phi)}\}$
Intensity,

$I=I_0\left|e^{i\omega t}\right|^2\{ 1 + e^{i\phi} + e^{i2\phi} + e^{i3\phi}\} \{ 1 + e^{-i\phi} + e^{-i2\phi} + e^{-i3\phi}\}$ $\left|e^{i\omega t}\right|^2=1$,
$\{ 1 + e^{i\phi} + e^{i2\phi} + e^{i3\phi}\} \{ 1 + e^{-i\phi} + e^{-i2\phi} + e^{-i3\phi}\}$
$=$$\frac{1-e^{i4\phi}}{1-e^{i\phi}}\cdot\frac{1-e^{-i4\phi}}{1-e^{-i\phi}}=\frac{2-e^{i4\phi}-e^{-i4\phi}}{2-e^{i\phi}-e^{-i\phi}}$
$=$ $\frac{2-2\cos{4\phi}}{2-2\cos{\phi}}=\frac{1-\cos{4\cdot 2(\frac{\phi}{2})} }{1-\cos{\cdot 2(\frac{\phi}{2})} }$
$=$$\frac{\sin^2{4\frac{\phi}{2}}}{\sin^2{\frac{\phi}{2}}}$
substitue $\phi$

$I=I_0\frac {\sin^2{\pi(\cos{\theta}-1)}} {\sin^2{ \frac{\pi}{4}(\cos{\theta}-1)}}$ (1)

Notice

Above eq.(1) is valid when $e^{i\phi} \neq 1$ i.e. $\phi \neq 0$
When $\phi = 0$, $I=16I_0$ from orignal Intensity eq.

second method

just using eq.(30.3)

$I=I_0\frac {\sin^2{n\frac{\phi}{2}}} {\sin^2{ \frac{\phi}{2}}}$ substitue $\phi$, $n=4$

$I=I_0\frac {\sin^2{\pi(\cos{\theta}-1)}} {\sin^2{ \frac{\pi}{4}(\cos{\theta}-1)}}$

graph

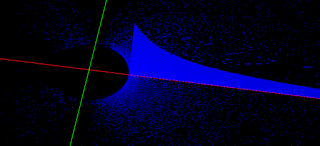x: red, y: green
Initial intensity :1
Intensity scale : 1000
distance > 100

---

This could be wrong.